Amanda and I just finished the scavenger hunt, big thanks to everyone who helped us (which was a lot of people). We’ll post all our clues later!
If anything, this scavenger hunt made me realize that I cannot do math by myself, especially under a time limit.
And that math majors are super nice and always willing to help, even if you ask them a random calc II question in the middle of the green bean.
It’s the last Thursday of classes, and I only have one class left for the semester, which thankfully is FOM. I’ll be on the other coast next semester at California State to fulfill ELAW, so I won’t be back on the river for something like 8 months.
I’m the type of person to embrace change, but dang, I will really miss the river and this campus.
Okay so I have this Comparative Economic Systems final in 13 days. It’s 25% of my grade, and cumulative. I basically have to know everything about capitalism, socialism, and the economic framework and history of 7 countries to the point where I could compare and contrast any aspect of them with any other country.
So instead of starting to study the insurmountable amount of information I’m required to know, or finding more clues for the scavenger hunt, I’m just going to prove that thing we talked about in class on Monday.
Using the definition of limit given, prove:
lim (x→0) x² = 0
So the first clue has something to do with marbles and distributions. Amanda figured out that it has something to do with Pascal’s triangle, where Pascal’s marble run distributes the marbles in a bell shaped curve.
Also when I googled Pascal’s Marble Run this picture came up:
Is this what we’re looking for??
Because who doesn’t want to compliment someone/pick someone up while showing off their knowledge of mathy math?
The above quote is one of the many reasons I love FOM/Casey. Anyways, Casey wanted us to work on proving a theorem as part of our homework this weekend, and I am 75% I figured it out last night.
Cantor-Bernstein-Schroeder Theorem:
If ∃ injective functions f : A → B and g : B → A, then ∃ a bijection b: A → B.
Okay, so first of all we’re assuming that those two injections exist, and we’re trying to prove that those two injections mean that a bijection exists.
For f : A → B to exist, there must be at least as many outputs as there are inputs so that f(a)=f(b) ⇒ a=b can be true. If there were more inputs than there were outputs, every input would not have a unique output, and would therefore not be an injection. So, based on this |A| ≥ |B|.
But, there exists another injection g:B → A, which means that |B|≥|A| based on the same reasoning above.
For both of these functions to be injective, we need to combine |A|≥|B| and |B|≥|A|, which means that only way both injections could be true is if |A| = |B|.
For a function to be bijective in between two sets, they have to satisfy onto and one-to-one. For one-to-one, as said before, there needs to be at least as many inputs as outputs. There can be more outputs not mapped to with injectivity. But with onto, all outputs must be mapped to, so there must be at least as many inputs for all outputs. This means that for a bijection to exist, |A| must equal |B|.
Hey! We just proved that it does if there are two injections in existence!
I think this proves this, right? Word. Happy Saturday ya’ll.
Blah blah blah, yes I talk about Micro a lot.
But these two classes have been overlapping like crazy to me lately. They’re both using ambiguous models that tend to confuse the crap out of me.
In micro, we’re talking about oligopolies. Oligopolies focus on the most important firms in a market. It’s pretty much in between perfect competition (with a lot of firms fighting for consumer money) and monopoly (with one big guy making production decisions), but it’s categorized more towards the monopoly end of the spectrum.
Like FOM, Micro uses a lot of models for more of a self-reference tool to see how economics works in theory, only to apply it to real world situations later. The three models we’re talking about right now are the Cournot Model, the Bertrand Model, and the Stakelberg Model.
With every economic (or other any field really) model, you’ve got to assume some things first. This model keeps it simple, and only keeps up with two firms in the market, which is called a duopoly. You’ve also got to assume that each extra unit of output produced costs the companies nothing (Marginal Cost = 0; but the model can compensate for positive, constant marginal coast if need be). The last assumption is stretching a little, but it’s crucial to make this model work. We need to imagine that each firm assumes that the other (it’s rival) will continue producing at it’s current level.
Basically this model shows how each firm will react to it’s rival’s output, through two reaction functions. Through brutal algebra, we can take a total demand function and marginal cost, and tell you how much each firm will produce, what price they’ll charge, and even how much money they’ll probably make out of it.
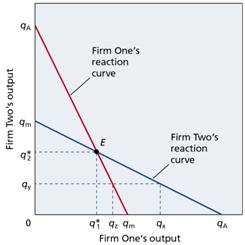This graph shows the stable equilibrium of a Cournot duopoly. At point E, there is no need to react any differently and revise output, because both firms are acting according to the other’s assumptions. This equilibrium is called the Nash equilibrium, named after a really cool (but crazy) math/econ dude. If you have not seen A Beautiful Mind, you need to.
The Bertrand Model is much simpler, and makes most of the same assumptions the Cournot model makes, but imagines that firms are reacting off of announced prices.
Say Firm 1 announces their price on a product. Firm 2 has three options. They can charge a higher price, and sell nothing. They can charge the same price, and split the market with Firm 1. OR, they can charge a lower price, and capture the whole market. Both firms obviously want to make the most amount of money, so both of them strive to undercut prices just low enough to make some money, but to be lower than their rivals price (think price matching at grocery stores).
This actually works against both of them. Both firms will end up cutting prices so low, that price will be set equal to the amount it took to make that one extra unit (MC), and the firms will split the market.
Silly firms.
The Stackelberg Model is probably the most realistic of all three models. It considers two models, but takes into consideration that one firm is more experienced than the other. We treat the follower’s output level as given, and substitute the follower’s reaction function into the market demand equation to find the leader’s output.
This makes sense intuitively, because the follower firm is really the only firm doing the reacting. The leader firm is the one making the big decisions.
The take away message from these three models is very simple: the more competition you have in a given market, the more output and lower prices you’ll get. Competition is a good thing, people.
Anyways, these models all seem simple enough, but when put to the real-life application test, they seem to break apart. You can’t just assume that companies will act rationally.
I’m just thinking about all of the high-school algebra I have to work through to make any three of these models work for my Micro final.
We had a huge discussion in FOM today that brought to light some of the concerns I’ve been having with numbers lately. We talked about Euclidian Axioms, and how basically, that’s just one model that we use to reference numbers and the world around us. And get this. Basically, the type of math we use is still a working model. Some brilliant, John Nash-type could wake up tomorrow morning and find a contradiction in the math we’ve all grown to know and love.
Our best defense against it? Whenever a contradiction comes up, we go back to the axioms, and make sure there are no errors.
No offense, but that sounds a lot to me like bringing a knife to a gun fight.
This also made me think about what we’ve been proving lately in class. We went over one horse theorem in class, with a proof that proved that all horses are the same color. One subtle detail made the proof fall apart. We ended up pulling it apart because they intersected two sets – and why would you do that?!
But then I’m thinking back to another complicated set Casey showed us in class, where he did some random mathy thing (forgive me for not remembering), and said something basically to the effect of, it works, and took someone a really long time to figure out that this proof works this way, so yeah.
I see differences in these two situations obviously, but they are sort of similar to me as well. What makes math work? Does anyone really know?
I think more than ever I’m realizing that math is 20% number crunching, 5% knowing what you’re doing, and 75% acting like you know what you’re doing.
This is sort of eye-opening for me, because I’ve loved math all this time because of the lack of ambiguity.
FOM right now is just making me really philosophical. I don’t think I can handle this much longer, man.
Since I’m a total sucker for helping people out and supporting causes, I figure I’d let everyone know that it’s One Day Without Shoes today! I bought my first pair of TOMS 4 years ago, and since then have provided 7 kids with shoes (one for one) through buying cute things, and given 1 person sight through buying a pair of their sunglasses. I know there are lots of causes out there that deserve consideration, and while I am not technically participating in ODWS this year (still rocking the apparel/shoes though!), I can tell you from past years’ experience that living without shoes for a day is extremely difficult and painful.
Imagine what it would be like if you didn’t own shoes. Or clothes. For your whole life.
I don’t know, just something to think about.
To learn more, watch this video! It’s cool.
As per the second to last Monday of the semester, I’m thinking over the past 12 or so weeks, and wondering if I’m any smarter at this math stuff. Casey did say we’d learn how to prove things in his class, but am I there yet?
I’m not going to lie, I really thought I would take this class, and somewhere right in the middle of the semester we’d come up with this universal proof writing formula that could be used for all mathy things, everywhere.
What I’m realizing ties back to the quotes we blogged about at the beginning of the semester (corny I know). Math isn’t all formulas. It requires common sense, and the sense to throw that common sense out the window when necessary (ie thinking about infinity). Math is what you make it, and for me it’s still fun. Here’s what I’ve learned so far:
Maybe FOM is making us smarter, even though it feels pretty confusing mostly all of the time.
FOMP WOMP. 